Break Apart Strategy in Math

Do your students struggle with adding and subtracting 2-digit and 3-digit numbers? The break apart strategy can make math easier and lead students to add and subtract mentally. Here we’ll explain why the break apart method is effective and how to best teach the strategy in addition and subtraction.

What is the Break Apart Strategy in Math?

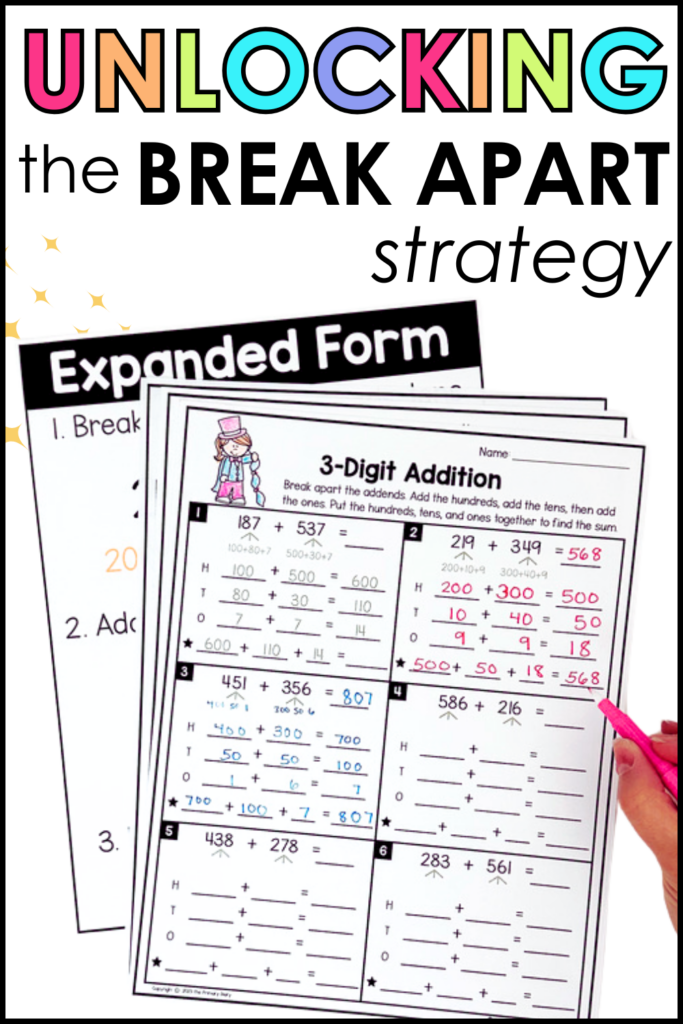
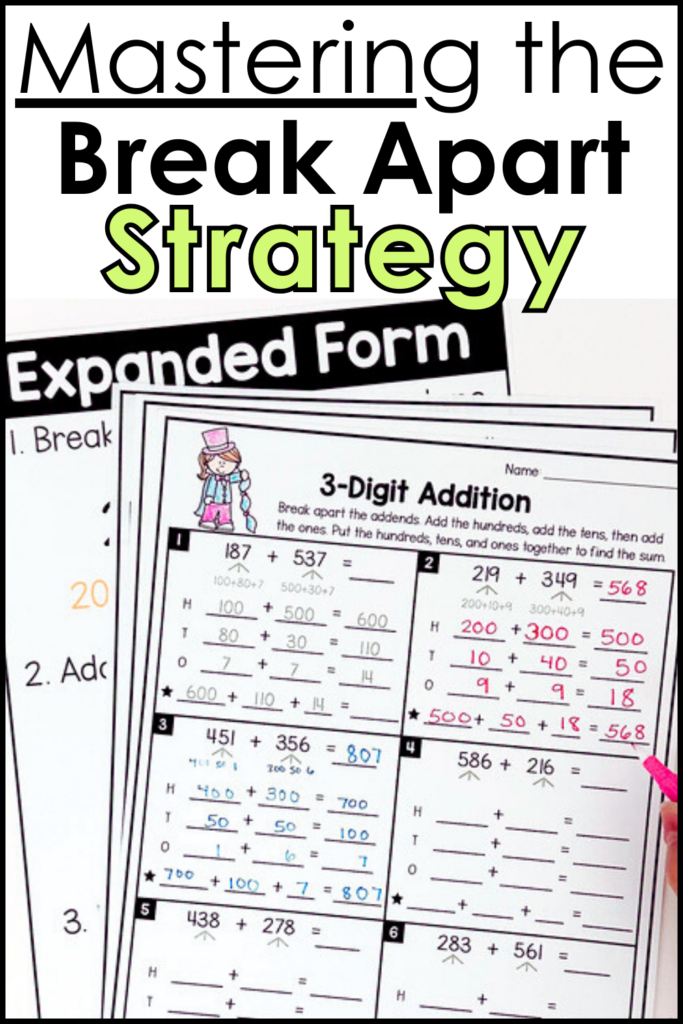
The break apart strategy is a method of breaking apart numbers into their place values. Then, either separating (subtraction) or putting together (addition) by place value to arrive at your answer. (e.g., breaking 36 into 30 + 6).

This method is preferred over the standard algorithm because students demonstrate their knowledge of numbers. For example, the number 356 is 300, 50, and 6, not a 3, 5, and 6. Using the break apart strategy supports mental math! Ask an adult how they would solve a 2-digit addition problem. Chances are they will do some form of composing and decomposing of the tens and ones!

How to Teach the Break Apart Strategy for Addition

Step-by-Step Guide:

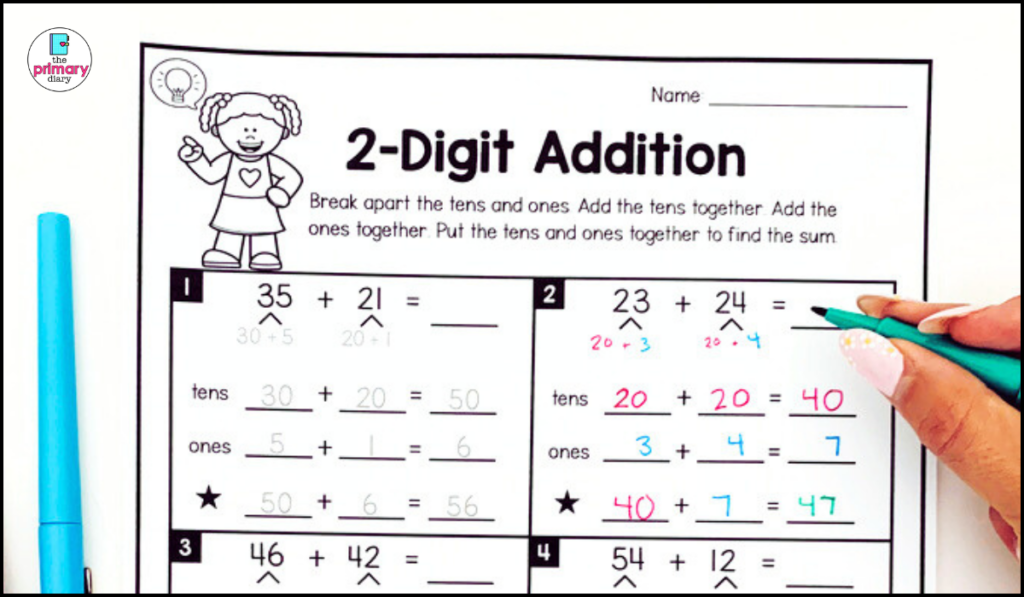
1. First, break down numbers into place values (i.e., 48 + 35 becomes 40 + 8 and 30 + 5).
2. Group the tens, then the ones. Following this example, 40 + 30 = 70 and 8 + 5 = 13.
3. Combine your tens and ones. In our example, you will need to regroup the 10 in 13. You now have 40 + 30 + 10 = 80 plus the 3 ones. 80 + 3 = 83.
4. This method is the same for 3-digit numbers, but you now have hundreds, tens, and ones to group!

Classroom Tips:

• Assigning a color for each place value helps students visualize the pattern—plus, using colored pencils in math is so fun!
• Hang an anchor chart on your math wall for students to refer back to.
• Scaffolded worksheets with examples provide a point of reference for students.

Using the Break Apart Strategy for Subtraction

Like with any method, subtraction can be trickier for students! For the break apart strategy in subtraction, you only need to break apart the subtrahend, or the second number in the subtraction problem. Here’s how to help:

1. Introduce the strategy by discussing a story: 72 – 45 (e.g., there are 72 students in 2nd grade; 45 went out to recess).
2. First, break the subtrahend into its place values (40 + 5). Keep the 72 whole. This is our starting number or total.
3. Next, subtract the tens from the total (72 – 40 = 32).
4. Last, subtract the ones (32 – 5 = 27).
5. The steps are the same for 3-digit numbers, but with hundreds, tens, and ones!

Teaching Tips:

• Practice with simpler problems before progressing to more complex ones. For example, start with problems without regrouping (e.g., 75 – 3) before moving on to regrouping problems (e.g., 75 – 8).
• Send a parent letter at the beginning so that they can support their student at home. Parents learned math differently, so they appreciate knowing how to help their child be successful.

Conclusion

Teaching your students the break apart strategy in math will lay a strong foundation for place value. With repeated practice, you will find students composing and decomposing numbers mentally.

Ready to get started? You’ll find everything you need to get started with the 2-3 Digit Break Apart Strategy on my TpT here: parent letters, posters, and step-by-step practice so that your students will find success.

I’d love to hear how it worked in your classroom!